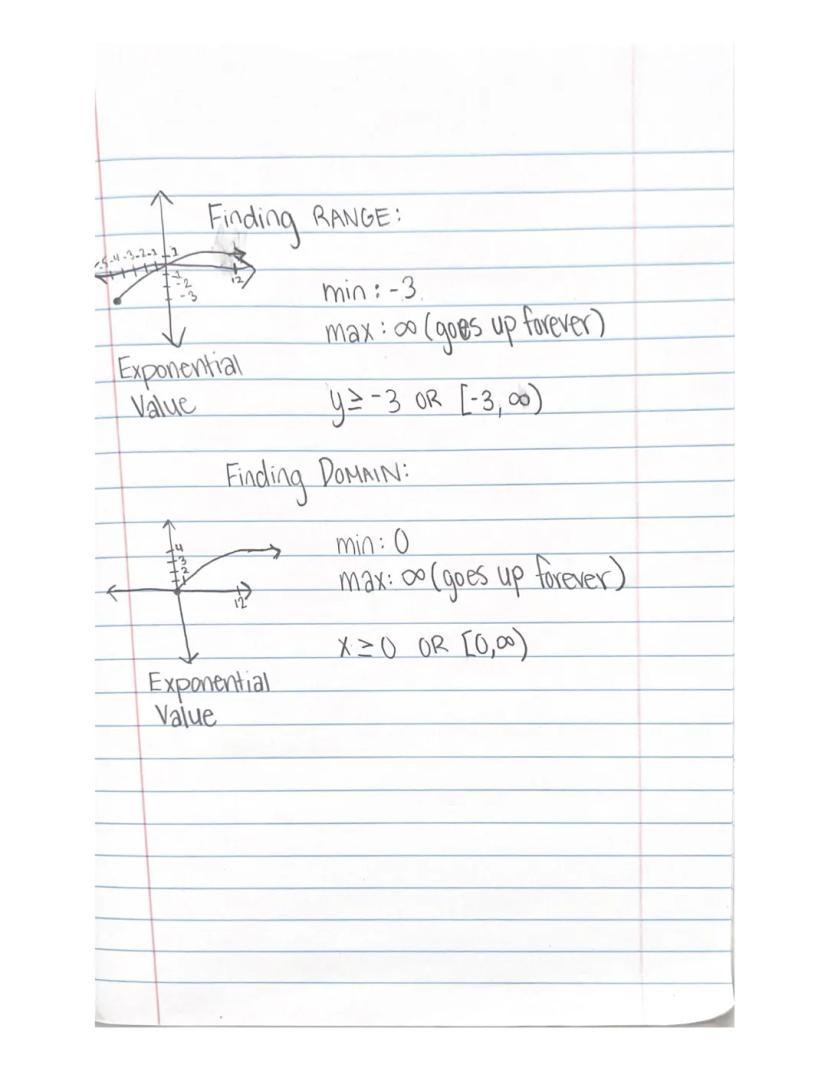
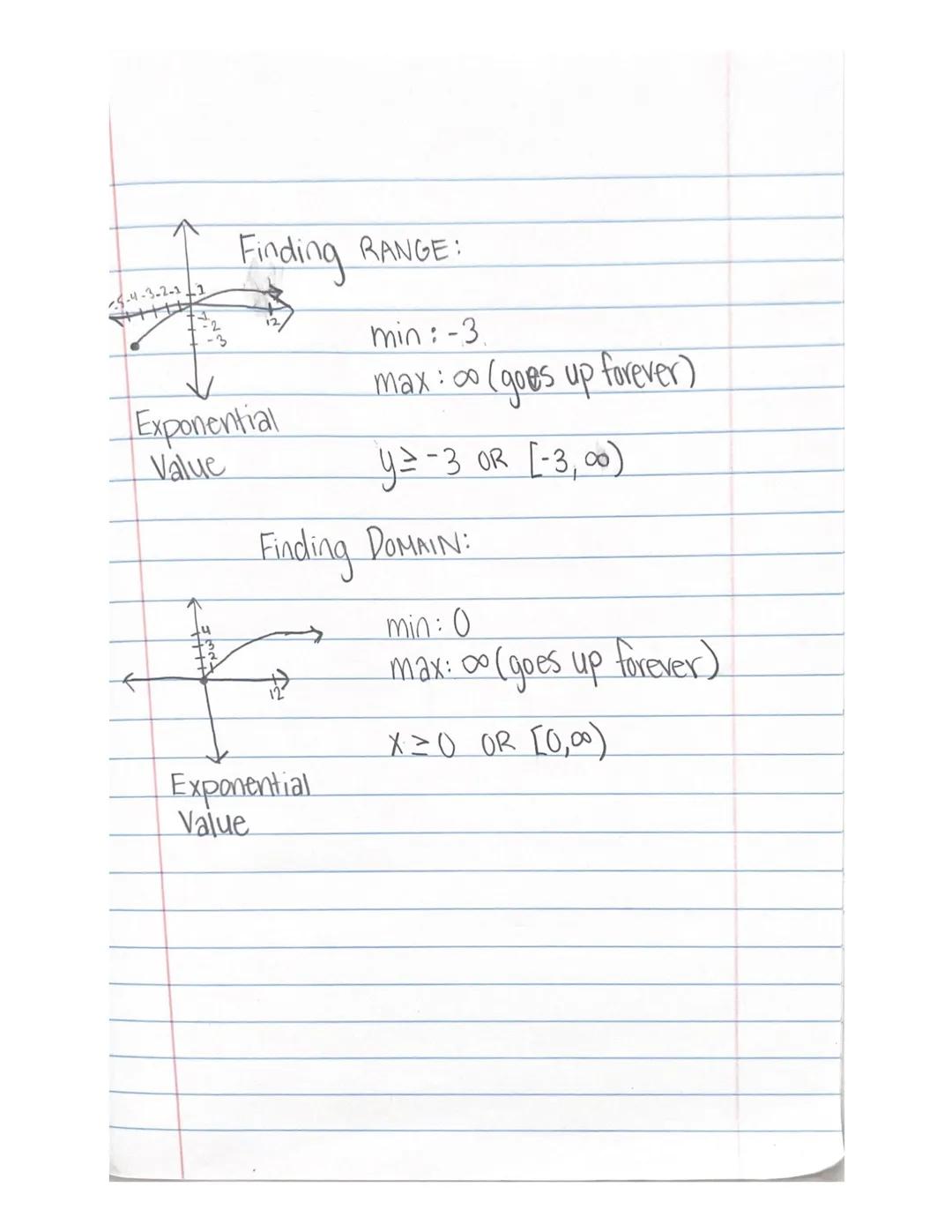
A comprehensive guide to understanding domain and range in mathematical functions, focusing on quadratic and exponential relationships.
- The domain represents the set of possible x-values (horizontal axis) that can be input into a function
- Range encompasses all possible y-values (vertical axis) that result from the function
- Notation can be expressed using interval notation with square brackets for closed intervals and parentheses for open intervals
- Special attention is given to quadratic function domain and range explanation and exponential functions
- Key concepts include understanding infinity notation and identifying closed versus open intervals