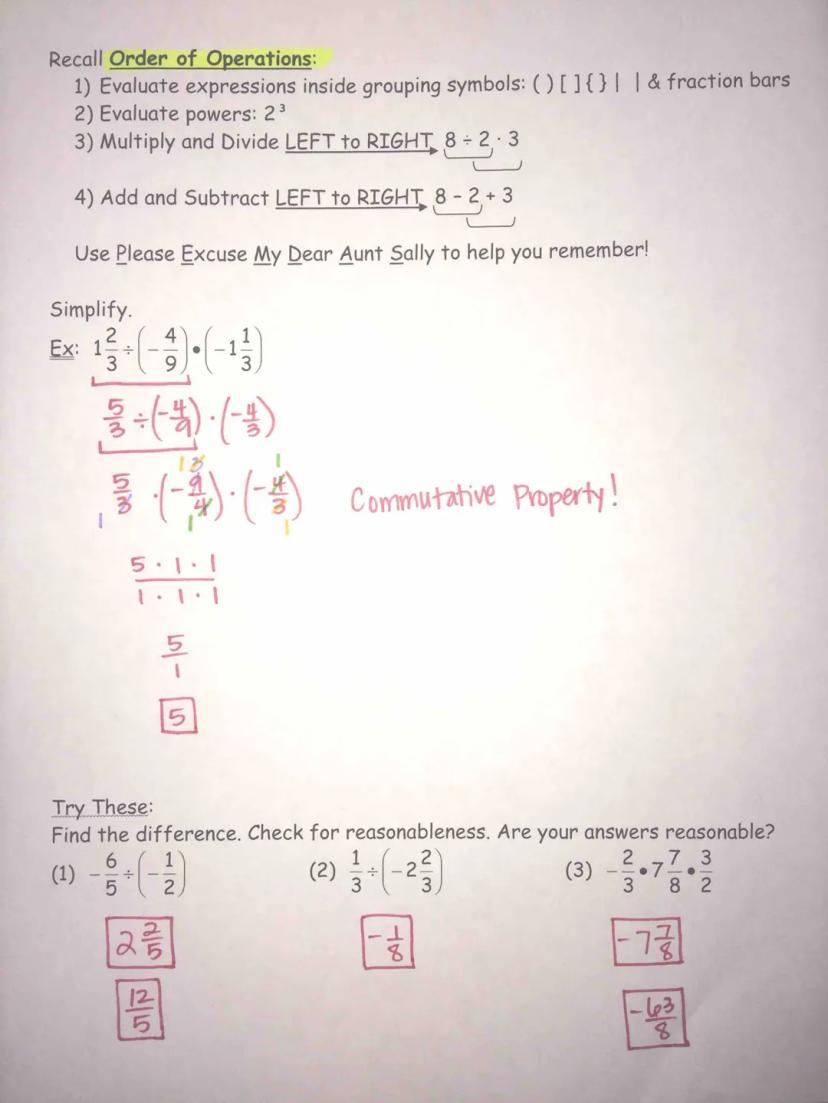
Multiplying and Dividing Rational Numbers - Fractions

This page introduces the fundamental rules for multiplying and dividing rational numbers, specifically focusing on fractions. It covers the sign rules for multiplication and division of integers, which are essential when working with negative fractions.

The page explains the process of multiplying fractions, which involves multiplying the numerators and denominators separately and then reducing the result if possible. For division, it introduces the concept of using the reciprocal of the divisor.

Definition: The reciprocal of a fraction is obtained by flipping the numerator and denominator. This is also referred to as the "re-FLIP-rocal" in the text.

Several examples are provided to illustrate these concepts:

Example: -²/₇ × ³/₅ = -⁶/₃₅

This example demonstrates multiplication of fractions with different signs, resulting in a negative fraction.

Example: ¹⁷/₄ ÷ ¹/₃ = ¹⁷/₄ × ³/₁ = ⁵¹/₄

This example shows division of fractions by multiplying by the reciprocal of the divisor.

The page also touches on important mathematical properties:

Highlight: The commutative property (changing order), associative property (changing grouping), multiplicative inverse (multiplying by reciprocal equals 1), and multiplicative identity (multiplying by 1 equals itself) are all mentioned as key concepts in fraction operations.

These properties are crucial for understanding more complex fraction operations and for simplifying expressions efficiently.